Here is an example that shows how much conventional measurements between points positioned by GPS vectors can improve the relative positional uncertainties between points. That is of course the key test of whether a survey meets the ALTA/NSPS specification for survey accuracy. This is a part of a larger project that I'm working on, but it works as a standalone example.
The network was surveyed by GPS vectors first and then conventional traverse measurements (horizontal angle, distance, zenith angle) were made to connect adjacent control points in the process of locating various other non-GPSable points along the traverse. Here's the basic diagram. It's a part of the project of fairly limited extent: 355-12 = 4861 ft.
The connection to NAD83 was made via OPUS solutions from sessions on several days at Control Point 355 and connections were surveyed from 355 to CP 1 and from CP1 to CP 12. The other points were surveyed by GPS vectors from CP1 and CP12.
The results indicated that the following were realistic uncertainties of the NAD83 coordinates of the points 1 through 355:
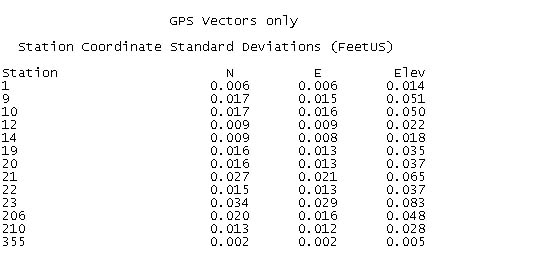
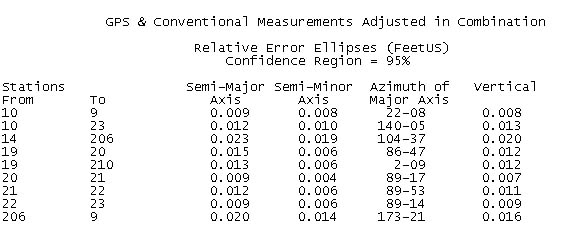
Looks cool, right? The problem is seen when the relative positional uncertainties (95% confidence relative error ellipses) between adjacent points are evaluated. By inspection, many of these are either too large to meet the ALTA/NSPS accuracy spec or are right at the edge of failure.
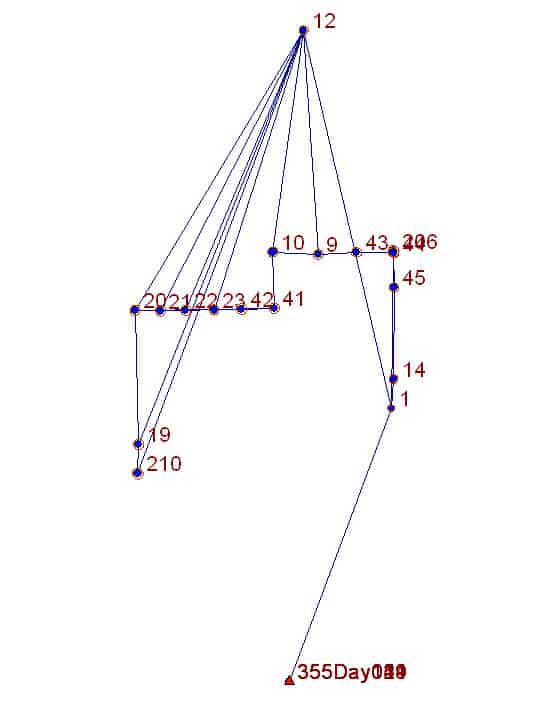
However, I also ran a conventional traverse through the control points (which in any event was necessary to make ties to various non-GPSable points along the way). Those conventional measurements were adjusted in Star*Net together with the GPS vectors weighted by their covariances. Here is the GPS-only diagram with the conventional connections added.
What effect did that have? The standard errors of the horizontal components of coordinates were improved a bit, but not drastically, the height components were improved considerably.
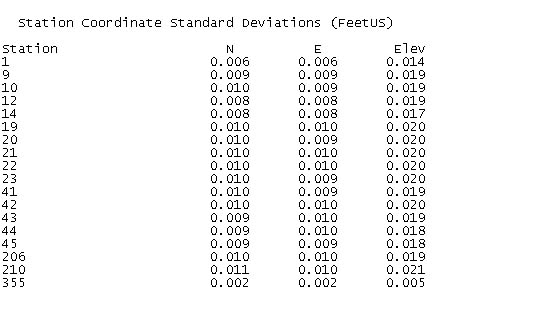
The relative position uncertainties of adjacent control points, however, were reduced by quite a bit and obviously all meet the ALTA/NSPS specification by a wide margin. Naturally, the vertical uncertainties show the greatest improvements as the points were connected by reciprocal zenith angles to derive height differences.
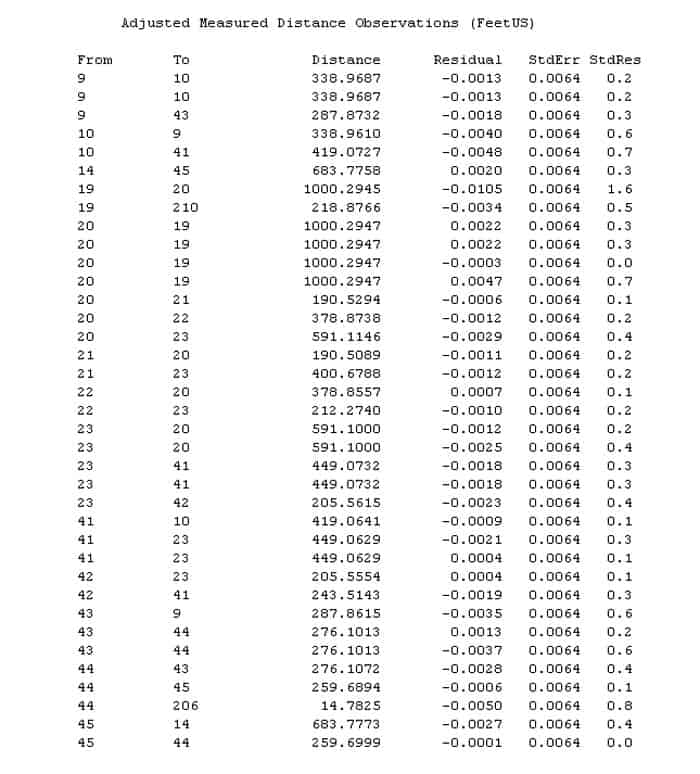
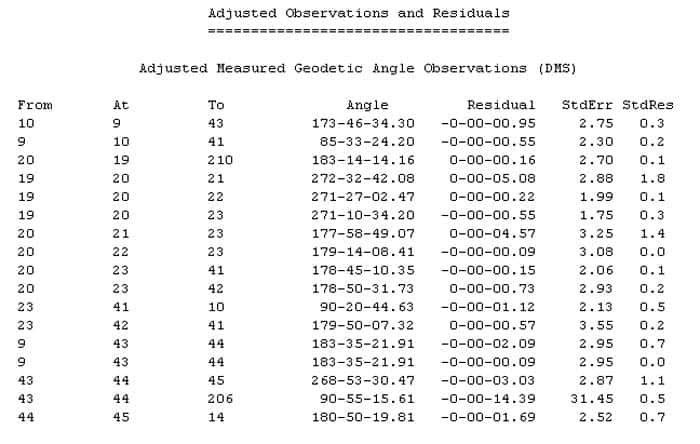
By the way, the conventional traverse was run with fairly ordinary methods, a Sokkia CX-105 with laser plummet for centering and Sokkia tilting prisms and targets on SECO prism poles (with 8-minute vials) centered in SECO prism pole tripods. Here is what the residuals looked like when the traverse was adjusted in combination with the GPS vectors: