-
LSQ, Transit, Compass, Crandall Adjustments
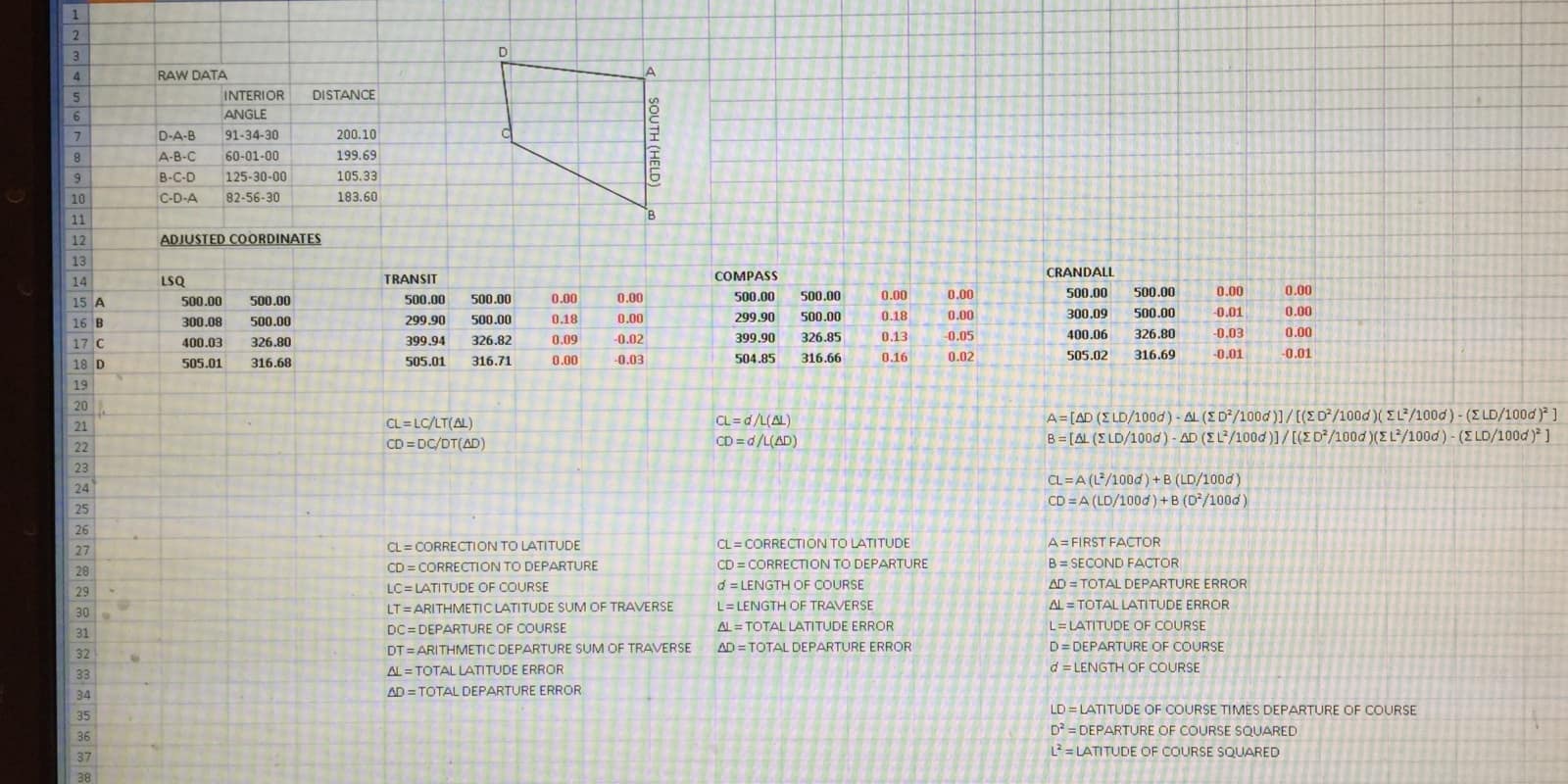
After decades of being a surveyor, I finally compared adjustment results between these four methods.
I must have been pretty busy….The red numbers are the LSQ solution minus the “arbitrary method” of choice.
-
9 Replies
-
Scott,
Not a useful exercise as you have it laid out.
I would suggest first starting with the raw coordinates, i.e. 5 coordinate sets, then the various adjusted coordinates. Between or beneath the coordinate sets should be the adjusted angles and distances. That gives you a complete idea of what has happened and you have more apples to compare with your apples as well as oranges to compare with your oranges. You want to be able to see how each adjustment worked differently.
Since the raw angles misclose by 2′ and the least count on angles is 30″ this suggests an ancient transit survey. A valid transit traverse should have included 3D and 3R readings added on the plate. When the final number for each set is divided by 6, I would not expect results to the nearest 30″. This may well be a single observation or just a D&R.
I suggest a “Paul’s Modified Compass Rule” adjustment.
1/ Adjust all angles by 67% of the angular closure error, in this case it would be minus 20″ at each corner.
2/ Then apply the compass rule adjusting distances.Your distance corrections should not be so brutal and your final coordinates should be comfortably close to your least squares solution. With a modern total station my method comes very close to LS, with the greatest error in the middle. Cross ties would greatly improve my method, but ties are even easier to now include with LS.
I would be at colleges teaching my method, but some durn fool invented computers making an actual least squares solution so easy that even the least qualified surveyor can do it. I guess I was just born behind my time.
Paul in PA
-
In college, many times the professor would give a lecture and at the end of that, he would mention that further study on the subject would be necessary for us to understand the details.
That was his way of letting us know that was all he was going to say on the subject and on our own would dwell deeper into the subject matter.
Each method has its own unique purpose depending upon the probable cause of error and where the user places confidence in either the distance or angle values.
I always would take the closure and find the most obvious setups where most of the errors were possible to be by bisecting the closing vector and looking at those setups located along the resulting perpendicular vector.
Another visit to those points may be in order depending upon the error values.
Once that is satisfied, I would balance the angles and check the closure again.
When that resulted in a much better closure, I would use the Crandall method.
We did have one crew that did really bad angular work and I would use Transit or Compass for best results.
From your findings, the Crandall is mostly aligned with LSQ results.
All these methods are fine.
I believe that everyone should spend the time to do a long hand DMD computation of a traverse or boundary calls to really appreciate the science behind the methods.Thanks for sharing…….
-
In looking at a plot of the raw data I find something else troubling. Line D-A closing is almost parallel to but South of beginning line D-A. 0.5′ and 0.4′ and the end point of closing line D-A is 0.4′ East. In looking at the raw data a transposition of the field noted 199.69′ to 199.96′ takes care of about 1/2 the closure error.
Also the distance A-B could be a misreading of the tape, adding a tenth for 200.1′ rather than subtracting a tenth for 199.9′.
LS has made most of the corrections on those two lines.
One has to consider the probable tools used, a 1′ or 30″ transit and a steel tape with solder marks only at the feet and tenth marks at 0 to 1′ or more likely 0 to minus 1′.
Before doing any adjustment one must first look for blunders and transpositions.
Fixing the transposition then adjusting appears appropriate.
Paul in PA
-
A Harris, post: 346840, member: 81 wrote: In college, many times the professor would give a lecture and at the end of that, he would mention that further study on the subject would be necessary for us to understand the details.
That was his way of letting us know that was all he was going to say on the subject and on our own would dwell deeper into the subject matter.
Each method has its own unique purpose depending upon the probable cause of error and where the user places confidence in either the distance or angle values.
I always would take the closure and find the most obvious setups where most of the errors were possible to be by bisecting the closing vector and looking at those setups located along the resulting perpendicular vector.
Another visit to those points may be in order depending upon the error values.
Once that is satisfied, I would balance the angles and check the closure again.
When that resulted in a much better closure, I would use the Crandall method.
We did have one crew that did really bad angular work and I would use Transit or Compass for best results.
From your findings, the Crandall is mostly aligned with LSQ results.
All these methods are fine.
I believe that everyone should spend the time to do a long hand DMD computation of a traverse or boundary calls to really appreciate the science behind the methods.Thanks for sharing…….
Andy,
When you do a few of these by longhand you really begin to see the dedication to good work some of the old surveyors like P.J. Godeau had -
Many LS solutions are possible by altering the standard error estimates. We hope they fit the actual field accuracy of distances, centering, and angles. What values did you use?
. -
What struck me right off was Professor Charles Crandall’s treatment of the issue.
Compass rule obviously was meant to fix compass and tape surveys. And the transit rule, transit and tape surveys.
But Crandall sought to provide a Least Squares simulation for ease and accessibility in an age of low tech (i. E. 1908ish).If I was a young chief without the means of a CADD system or expensive LSQ software package I’d want to be able to do the Crandall method on my traverses.
-
In my opinion using LSA doesn’t make significant differences unless you have surveys with many “cross ties”. With a loop traverse there really isn’t enough redundancy for there to be a great deal of difference and the difference is also hard to evaluate. For LSA of networks with many cross ties and lots of redundancy most programs adjust using either condition equations or observations. Regardless it seems to me there aren’t any assumptions about the relative accuracies of angles compared to distances. The assumption is simply that the apriori error estimates of angles, distances, target heights, centering etc. are realistic. That they realistically reflect the amount of error that will be observed in these observations. This seems to work great with conventional angle and distance measurements, but, incidentally, doesn’t work all that well with GNSS measurements.
Having said that I do remember having problems years ago with Compass adjustments and switching to early LSA methods for that reason. We were doing very high accuracy traverses in metro tunnels with T2’s and calibrated EDM, prisms etc. We were observing eight positions on the horizontal angles and most of the traverses were string traverse from two control points, down a tunnel to close on two more. A Compass adjustment was putting much more error in the starting and closing angles than was really there in the measured angle. If you measure eight positions with a T2 you have a pretty good idea what the limits of the error in the angle really are. In other words we were getting angular spreads of a few seconds yet the Compass rule was putting much more error in those angles than what we had measured. After switching to an early NGS LSA (TRAVERSE) program that used condition equations the results got much better. By that I mean the opening and closing angles were adjusted a much smaller amount and about the same amount as the other angles, so the problem wasn’t in the error in the coordinate values of the control points but simply in the method of adjustment.
-
I would like to say that the Compass Rule should be called by its correct name ” Bowditch Rule”.
This is what Joseph “Joe” Dracup of NGS in 1973 had to say: ” The Compass Rule is called in most parts of the world, the Bowditch Rule after Dr. Nathaniel Bowditch who first stated it. Dr. Bowditch was an American and hopefully one day the word “Compass” will be replaced universally with his name whenever reference is made to this rule”.
ref. Surveying Instrumentation and Coordinate Computation Workshop Lecture Notes, American Congress on Surveying and Mapping, Control Surveys Division, 1973, page 102
I guess that not much progress has been made in 42 years.
JOHN NOLTON
Tombstone, AZ -
Does it really matter which adjustment is used on a four sided figure with and angle error of 2 minutes? LS is the best adjustment on a survey with adequate redundancy. There is no adjustment that can make chicken salad out of chicken manure. I use LS for all traverses. I do also look at the angular closure and error of closure along with the statistical analysis. The last time I accepted 30″ per setup was using a 1 minute transit and didn’t like it then.
Log in to reply.